"Scientists are peeping toms at the keyhole of eternity"
Arthur
Koestler (1905-1983)
The Roots of Coincidence
Electron Spin of
1. Introduction
The objective of physics is a study of nature. However, the former 20th century has passed mainly under the guise of ascribing fictitious attributes to nature directly following Einsteinís approach (where "was a great free of the imagination: a great space for self-chosen assertions about what will ultimately work" [David Bodanis, E=mc2: A Biography of the Worldís Most Famous Equation]). There are a lot of examples which confirm this statement. Here we present one of them devoted to the notion spin. The last was introduced in physics in connection with the theoretical error appeared at the mechanical description of Einsteinís-de Haasís experiment and based on the thoughtless approach at the derivation of average current of orbiting electron.
The average current was obtained on the basis of the mechanical model of uniform motion of the
electron regarded, in the classical spirit of the definition of current, as a flow of electric charge
("electron liquid") in a conductor. According to such a primitive mechanical model, disregarding
peculiarities of (1) wave motion and (2) closed motion along the circle, the average value
of orbital current, caused by the orbiting electron, unfortunately, has been accepted to be equal to the
incorrect ratio
 (1.1)
(1.1)
where Torb is the period of electronís revolution along an orbit. This
expression gave rise to all further fittings to the experiment.
By definition of the 1930ís, the magnetic moment of a closed electric circuit (in the specific case of
the electron orbit in the hydrogen atom) is assumed to be equal to
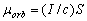 (1.2)
(1.2)
where I is the average value of current on the orbit, and S is the area of the
orbit. The relation 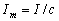 is actually the
circulation [1], called in physics the current in the magnetic system of units CGSM.
is actually the
circulation [1], called in physics the current in the magnetic system of units CGSM.
Resting upon the incorrect ratio (1.1) and the definition (1.2), the incorrect magnetic
orbital moment was obtained:
 (1.3)
(1.3)
which in turn led to the erroneous formula of the ratio of the electronís orbital magnetic
moment  to its moment of momentum
to its moment of momentum 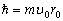 on the
Bohr orbit:
on the
Bohr orbit:
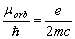 (1.4)
(1.4)
The value (1.4) is half as much the real ratio
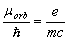 (1.5)
(1.5)
obtained experimentally by A. Einstein and De Haas [2-3]. Eq. (1.4) is inconsistent also with S.J.
Barnettís experiment [4, 5], etc. At that time, instead of seeking the error in the theoretical
derivation of the expression (1.4), the hypothesis-fitting was accepted. According to this hypothesis,
the proper magnetic moment 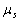 equal to the orbital
magnetic moment
equal to the orbital
magnetic moment 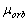 was attributed to the electron.
Further, naturally, in order to reduce in correspondence with the proper magnetic moment, the "proper
moment of momentum"
was attributed to the electron.
Further, naturally, in order to reduce in correspondence with the proper magnetic moment, the "proper
moment of momentum" 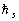 ("spin") of
("spin") of 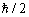 value was invented and attributed to the electron
as well. Thus, an appearance of correspondence of the theory to the experiment (Eq. (1.5)) was created
as a result of such a mathematical adjustment:
value was invented and attributed to the electron
as well. Thus, an appearance of correspondence of the theory to the experiment (Eq. (1.5)) was created
as a result of such a mathematical adjustment:
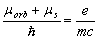 (1.6)
(1.6)
2. The correct approach
The erroneous formula of the average current (1.1) is a result of the product of the frequency of
revolution of an electron by the electron charge e: 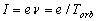 ; i.e., it represents the ratio of electron charge to the orbital period. Where is
the mistake? Let us turn to the analogy with a mathematical pendulum. Following the same logic, which
led to the formula (1.1), we should to announce the ratio of amplitude of displacement of a pendulum to
the period of its oscillations as the average speed of its motion, but it is absurdity.
; i.e., it represents the ratio of electron charge to the orbital period. Where is
the mistake? Let us turn to the analogy with a mathematical pendulum. Following the same logic, which
led to the formula (1.1), we should to announce the ratio of amplitude of displacement of a pendulum to
the period of its oscillations as the average speed of its motion, but it is absurdity.
The motion in inner space of a circular trajectory, along two successive half-circumferences, occurs in one direction (clockwise or anticlockwise) (Fig. 2.1a).
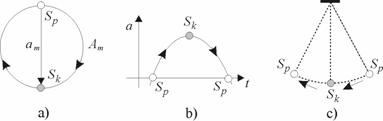
Fig. 2.1. (a) The amplitudes of displacement, am and Am, in a wave of the fundamental tone on a circumference; Sp and Sk are the potential and kinetic points (nodes) of the wave; the kinetic node represents the center of a loop of the wave (b). (c) A mathematical pendulum.
Simultaneously, the same motions in outer space, as mutually relative ones, are opposite-directed;
and one revolution of the electron is equivalent to one-half period (Fig. 2.1b) of pendulum swinging
(Fig. 2.1c). This fact shows the contradictoriness of the circular motion. If Sp is an
arbitrary potential point of a wave of the fundamental tone (i.e., its node), then, the
conjugated diametrically opposite point Sk will be the kinetic point of the wave (its
loop). The rectilinear amplitude of displacement is equal to the diameter of a circumference, 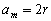 . The amplitude of the curvilinear displacement
along a circumference is equal to half-circumference, i.e., quarter-wave:
. The amplitude of the curvilinear displacement
along a circumference is equal to half-circumference, i.e., quarter-wave: 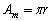 .
.
Let us consider the transfer of some property p (Fig. 2.2a).
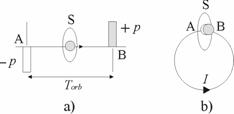
Fig. 2.2. (a) The exchange between the points A and B at a rectilinear part with the time interval Torb between them and (b) the exchange between the coinciding points, A and B, at the circular trajectory.
If during a period Torb, some property p is diminished from point A (analogous
to Sp in Fig. 2.1a) and the same property is arrived at point B (analogous to the same
point Sp in Fig. 2.1a), then the average speed of change of the property is
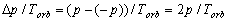 (2.1)
(2.1)
Thus, the transfer of any property of some object (a parameter of exchange p) will be
characterized by the average rate of exchange I, determined by the expression
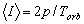 (2.2)
(2.2)
In the case of a circular orbit, when the points of exchange, A and B, coincide (Fig. 2.2b), an object
with mass m passes through the cross section S with the average speed of exchange
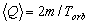 (2.3)
(2.3)
This value shows that during a period Torb, the mass of an orbiting object on the
right side of the cross-section S decreases along the value m and increases,
along the same value m, on the left side. Accordingly, the average speed of exchange of
the charge e in any section of circular trajectory is defined by the relation
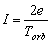 (2.4)
(2.4)
In reality, the orbital motion of the electron is the wave process represented by the cylindrical wave field [1]. Therefore, let us show the solution of the aforementioned problem on the basis of calculation of the wave motion of the orbiting electron, regarding the electron as a particle-wave. The electron (particle), being the discrete part of the wave, is represented by the wave node. (Other ways, leading to the same result (1.5), are considered in details in the book [1] and partly in the paper placed at this web site)
From the well-known solution of the wave equation for a string with the length l fixed at both
ends, 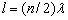 (where
(where 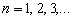 ), follows that one half-wave of the fundamental tone
), follows that one half-wave of the fundamental tone 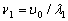 (where
(where 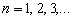 ), follows that one half-wave of the fundamental tone (where
), follows that one half-wave of the fundamental tone (where 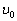 is the wave speed in the string) is placed on its
whole length l:
is the wave speed in the string) is placed on its
whole length l: 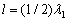 . If the ends of the string
are joined together, (where is the wave speed in the string) is placed on its whole length l: . If the
ends of the string are joined together, forming a string circle of the length
. If the ends of the string
are joined together, (where is the wave speed in the string) is placed on its whole length l: . If the
ends of the string are joined together, forming a string circle of the length 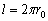 with one node, we have
with one node, we have
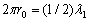 and
and 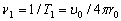 (2.5)
(2.5)
As shown in [1], similar to the case of the wave field of a string, only a half-wave of the
fundamental tone is placed on the Bohr first orbit, and the electron is in the node of the wave.
Because of this, the average value of current I, as a harmonic quantity, is determined by the
integrals:
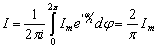 or
or 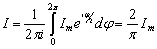 (2.6)
(2.6)
The amplitude of the elementary current is defined as
 (2.7)
(2.7)
where  is the frequency of the fundamental tone
of the electron orbit, equal to
is the frequency of the fundamental tone
of the electron orbit, equal to
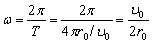 (2.8)
(2.8)
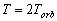 is the wave period. Hence, the average current of
the electron orbit is
is the wave period. Hence, the average current of
the electron orbit is
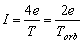 or
or 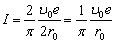 (2.9)
(2.9)
The above derivation, as all other ones described in [1], gives the average value of the current twice as large (1.1). Two (coinciding in our case) states of the beginning and the end of the period is the inherent feature of any periodic functions without which there is no period. And these states cannot be divided, as states of rest, by two parts belonging to two adjacent periods.
Taking (1.2) into consideration, we find the orbital magnetic moment of the electron, as the magnetic
moment of harmonic wave of the fundamental tone,
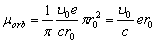 (2.10)
(2.10)
From this it follows that the ratio of the orbital magnetic moment of the electron to the moment of its
orbital momentum is
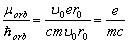 (2.11)
(2.11)
Just this formula is confirmed experimentally. It undoubtedly proves the inconsistency of the hypothesis
on the electronís spin of 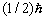 .
.
3. Conclusion
The spin myth gave birth to the theoretical spinomania. Of course, an electron has its own magnetic field
and magnetic moment and moment of momentum. But, as the calculations show [1], the last is
insignificantly small in comparison with the orbital moment. Let us imagine that the proper moment of
momentum of Earth is equal to one half of its orbital moment of momentum. The Earth cannot endure such a
huge moment and will be destroyed. The same situation will meet an electron with the "spin" equal to
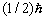 .
.
Diracís relativistic wave theory of spin (1928) [6], created for the proof of the correctness of an introduction of the spin of such a value, "proved" it. From that time, the further development has led to the electron being regarded, not as a real particle (which is unable to endure the huge moment which was ascribed to it), defined by three spatial coordinates, but as a top-like structure, possessing an angular momentum of its own. Dirac noted in this connection [7] that the aim is "not so much to get a model of the electron as to get a simple scheme of equations which can be used to calculate all the results that can be obtained from experiment".
As a result, due to the gross fitting, the formal correspondence of the "theory" with the experiment was realized. We state it resting upon the data [1], which convincingly show that Eq. (1.1) for the average value of current of the orbiting electron is erroneous. Accordingly, all equations obtained on its basis (including (1.3) and (1.6), etc.) are incorrect as well. For this reason, the Dirac equation is false and has significance only from the point of view of history of the philosophical and logical errors of the past. With that, in spite of the leading role of quantum electrodynamics in modern physics, one should not forget that the correspondence of any theory with the experiment does not quite mean that the given theory is true and uniquely possible. And what is more, the possibilities of modern mathematics are so impressive that it can represent any abstract absurdity as a profound theory (or its development) and fit it to the experiment. After all, physics must not only "calculate all the results" [7], but also comprehend Nature.
References
[1] L. Kreidik and G. Shpenkov, Atomic Structure of Matter-Space, Geo. S., Bydgoszcz, 2001, 584
p.
[2] A. Einstein, W.J. De Haas, Verch. Deutsch. Phys. Ges. 17, 152 (1915).
[3] A. Einstein, Verch. Deutsch. Phys. Ges. 18, 173 (1916).
[4] S.J. Barnet, Phys. Rev. 6, 171, 239 (1915); 104, 7 (1917).
[5] S.J. Barnett, Rev. Mod. Phys. 7, 129 (1937).
[6] P.A.M. Dirac, The Principles of Quantum Mechanics (third edition), Clarendon Press,
1947.
[7] P.A.M. Dirac, Classical Theory of Radiating Electrons, Proc. Roy. Soc., V. 168, p. 148
(1939).
George Shpenkov
January 9, 2003